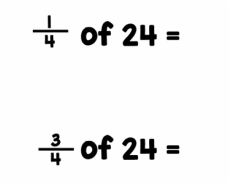
As we come to the end of the year, I've been thinking about how I've used number talks in my class this year. As I moved from Primary 1 (kindergarten) last year to Primary 3 (2nd grade) this year, it has been a bit of a challenge at times. Why? Because my understanding of mathematics is largely procedural. I can look at a problem and follow the algorithm I was taught years ago and get the right answer. BUT - I often have only a vague understanding of why the algorithm works. And I definitely struggle to 'think outside the box' and solve problems in ways I wasn't taught.

This year, I've found that I've needed to work hard (and pull out concrete materials to aid my own understanding of why a given strategy works!) to really understand what is happening when I use the multiplication algorithm, for example. Or how I can split numbers apart and combine them in different ways to mentally solve problems that the 2nd grade me would have definitely used paper, pencil and algorithm for.

​I think this is the main reason why number talks can be daunting for teachers at first - we are asking our kids to reason about mathematics that we might find just a little bit tricky ourselves (luckily, I've only been teaching Primary 3!). And what if the kids come up with a strategy that we can't follow, or that we can't make clear to the rest of the class? I benefited, I think, from starting the Number Talk process in kindergarten, so the different strategies I was getting my head around were simple.

​But when you teach this way, you really open up an exciting mathematical world for your pupils. As the year has progressed, I've often found myself thinking that some of my class have a much better intuitive grasp of mathematical fundamentals than I have (oops). They can reason with numbers in a way that often just never occur to me.

​Take as an example, the 2 fraction equations given above. I put the first equation to my class yesterday morning, and many of the kids were able to tell me that the answer was '6'. To help, I put up 24 different magnetic cubes, and we split them up into our 4 groups, so we could all see the 6 cubes in each group.

​Then we moved to the second equation. Again, a reasonable number of children could tell me that the answer was 18 (although this concept is definitely trickier for a lot of them!). But when I asked one little boy to defend his answer, this is what he told me:

​I knew that 1/4 of 24 was 6, and I had 3/4 left, so I took 1/4 (which is 6 cubes) away from 24 to get 18.

​Oh, My. Goodness. Of course - how simple. But it never in a million years would have occurred to me.

​Implementing number talks can be tricky. And you will definitely stretch your own understanding of mathematical processes as you implement them. But the rewards for your pupils are amazing, so I would really encourage you to give it a try. Start simple - even if you are teaching an older class, if they aren't familiar with number talks and having to explain their mathematical thinking, they will thank you (and you will thank yourself!) for starting slowly. But give it a try - you won't regret it.

This past year in Primary 1 (kindergarten), I've had dot cards and rekenreks readily available, so number talks have been easy to implement. I've realised, however, that I've done them randomly - I haven't followed the suggested sequences from the book.

I feel like the kids in my class benefited enormously from the number talks, and I was pleased with how their number sense developed over the year. Re-reading the book, however, I can see benefits of following the sequences given in the book - not least because it means I don't have to think on my feet nearly as much!

But it isn't very practical to have the book open next to you while you are teaching, so my daughter and I put our heads together to come up with a better way to approach this.

My very kind daughter photocopied all of the pages which give 2nd grade (Primary 3) number talk sequences. Each square you see on the page contains a sequence of 4 different equations which would be given one after another, to help the children focus in on using a given computation strategy.

We enlarged the page from A4 to A3 (not sure what that translates to on American photocopiers), to make the squares slightly larger. This summer, my daughter has been cutting out the cards for me, keeping each group of talks together (each page represents a group of talks). One page might be 1-2 weeks worth of number talks for your class, depending on how quickly they were picking up the strategy you were working on.

What to do next? Well, if you were to wander through my classroom, and have a look at the different resources available for the kids, you would quickly realise that I LOVE scrapbooking rings. So I obviously thought of them again when we were thinking about how to make it easy to sequence number talks effectively in the classroom.

Here, you can see the different sets of number talks that my daughter has cut out (all bundled together with rubber bands). Next to the unfinished sets is a set I've put onto a scrapbooking ring - I've ordered the cards in the same order given in the Number Talks book. The green card on the ring is my 'go' card - it lets me know that the following card is the first card in my number talk sequence.

Once these sets are all made up, I'll still have a bit of thinking to do. I suspect I'll need to add handwritten labels to the green card, giving the strategy those number talks are targeting. I'll also probably need to number them, so I have a rough idea of the general order (although I'm not above mixing things up a bit, if that seems like the right thing to do for my class!). Once we're further along in getting these ready for next year, I'll post more pictures and let you see how they've all turned out.